Mathematics as a discipline covers a vast body of knowledge and requires understanding of a wide range of principles, techniques and facts. Trying to memorize these by rote is in many ways a fool’s errand due to the extent of the task, but may also be fundamentally misguided. There’s an idea that highlighting something in a textbook is the easiest way of not remembering it. Kind of like how I said that telling somebody else your goals makes you less likely to meet them, highlighting something is an easy way of selecting something important and symbolically satisfying the need to acknowledge its significance. The brain is great at settling for “good enough,” and so it takes this as a signal to forget about the highlighted text until further notice*.  The same can happen with mathematical learning when you’re trying to memorize by rote, by repeating a phrase, or copying it out. I tried copying out some things from memory, and it’s more effective than trying to copy them out from the opposite page, especially if you keep coming back to them, but it’s not the right idea for much of mathematics.

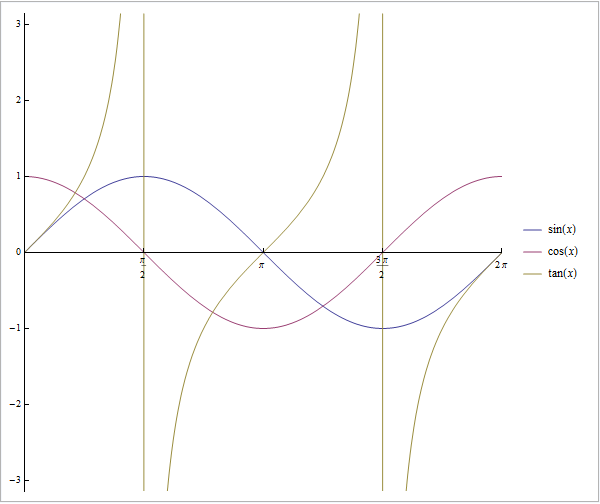
There are things called trigonometric identities, equations using angles inside triangles and telling us about the relationship between angles and the length of the sides. They’re incredibly useful and are fundamental to much of advanced mathematics, but there’s a lot of them and they can be hard to remember. One way of trying to remember them is to copy them out and then practice writing them from memory, using up a lot of paper, and probably having to stop and go back a bunch of times. At the end of it, maybe you’ve got them locked in, or maybe they’ll slip, but you probably won’t understand the significance of the ideas.

The thing about the trig identities is that they’re a hundred percent logical, and are based fully on much simpler principles. It can be hard to see the connection between the simple ideas and the more complex expressions of them, but working through the logical chain of reasoning can really help solidify not only your knowledge but your understanding of the identities. This is what I’ve been doing for the past few weeks, apart from teaching students, because I mostly teach year eleven maths and the trig identities are a little bit more advanced than what they’re doing, so I figured working through the proofs is a good way of establishing the underpinnings of the techniques they use in their geometric reasoning NCEA standard. The other useful thing is that once you understand the trig identities, you can see one version of an equation containing trigonometric functions and instantly, or at least quickly, see it or rearrange it as another, equivalent expression which might be simpler to operate with. This can really help with higher mathematics like calculus.

Sometimes, rote memorization is the only way, or it’s all you need. The times tables might be a good example, although personally I never learned them by rote. I’ve been using chunking and skip-counting instead, to relearn them and try to increase my speed. But I think, just like highlighting something and then forgetting it, theres an important idea that we could touch on later in another blog post. It’s about how the brain settles for “good enough.” There’s a saying, “practice makes perfect,” but I prefer to think “practice makes permanent.” If you practice something improperly, then you’re only reinforcing your improper technique, and the more you repeat, the worse it gets. This means experience isn’t necessarily the recipe for success. When you’re first learning something, it might be too difficult to perform the task properly, and so you find some approximation which actually makes the task slower, less efficient or ultimately more difficult, but which at the time operates as a short-cut. If you don’t, at some point, consciously unlearn this habit, then it can become strongly ingrained into your technique, and leave you handicapped for the long-term. So; let’s try and work logically, and slowly, through the problems we face, especially if they’re mathematical, and to understand why we’re doing each step. This way, our mathematical knowledge builds on itself, has strong foundations, and there aren’t any inexplicable gaps where we accept things “just because,” or idiosyncratic ways of completing some problems which might actually make them more difficult. Of course, this is simply an ideal, and generally impossible to meet fully in practice, but as a mindset it can be helpful to go back over things we think we’ve already got down, and re-examine our understanding or our assumptions, to make sure there’s not room for improvement somewhere. Periodic re-examination of our approach to difficult problems, and a willingness to unlearn bad mental habits, will stand us all in good stead.

*Theoretically, you’ll come back to the text and see what you highlighted, but that may or may not happen, and I’ve seen people highlight more than fifty percent of a page before, such that “if everything is special, nothing is.”

People who are good at remembering large amounts of stuff, like multiple digits of pi or whatever, tend to have interesting mental techniques they use to improve their skills. A couple of these, chunking and back-chaining, work well with the memorization of sequences. If you take a long series of digits and break it up into chunks, such that each small chunk has what seems like some kind of internal relationship, then you can memorize each of these chunks relatively easily. The working memory of the average person can only contain something like seven items, but if you break something up into chunks then each of those chunks can be one of the seven or so items you hold in your head at a time. If you can memorize the chunks, and then memorize the connections between them, you’ve got it down.

The problem can often be the connection between the chunks, where you’re reciting something and one chunk is complete but you’re unsure what comes next. I find back-chaining useful in this instance, where you look, not just at how things go forward, but how they go backwards, and practice connecting each chunk not just with the next but with the one before it. I’m currently using this and skip counting to relearn my times-tables.

Some of you from my generation or earlier might remember learning times-tables by rote in primary school. These days the curriculum is a little more broad and perhaps in some ways shallower, so if somebody misses their times tables we don’t like to necessarily punish them and so there might not be the same emphasis. This is probably good, in some ways, but it looks like there might not be the same facility with basic arithmetic in the children of today than yesteryear. Anyway; I never learned my times tables properly, back then. If I recall correctly, the learning process involved chanting “seven times seven is forty nine; seven times eight is fifty six.” I could do that fine, but I hadn’t actually memorized most of them, and instead was just figuring it out as I went along, because usually the time it took to state the complete sentence was enough to get the relatively simple calculation done.

But skip counting is a little different. We all probably know what that is, or at least we can recognise it when the skip size is two. It’s as simple as going “two-four-six-eight-…” up to whatever number. But it’s a little more complicated to go “three-six-nine-twelve-…” or “…twenty-one, twenty-eight, thirty-five…” with the various larger numbers. I find that, when I practice skip-counting with bigger skip sizes, I naturally start chunking the sequence, such that “fifteen-eighteen-twenty one” forms one chunk and “twenty-four, twenty seven, thirty” forms another. Sometimes, though, the connection between chunks isn’t as strong as the internal structure of the chunks themselves, which is where back-chaining becomes useful, going down through the sequence, and sometimes forming alternate chunk regimes, so that there’s a different way of breaking up the sequence which creates an overlap between the existing chunks.

The task I’ve set myself is probably relatively unimportant, but I like to keep my brain busy and have a few mental projects to work on just as a form of exercise. The process of chunking, however, is less trivial, and can be used as a great way to categorise, organize and internalize large amounts of information for efficient retrieval. Most of us do it instinctively to some extent, otherwise we’d never be able to remember anything of any significant size, and so that’s part of why books have chapters and songs have verses. But becoming conscious of the technique and finding new ways to apply it can probably improve our abilities in mutliple areas.

If you wanna improve your life, keep your goals a secret. Or at least, if you don’t, be warned that it may decrease your chances of actually meeting them. This seems somewhat counter-intuitive, and goes against some of the conventional wisdom and motivational advice out there. Especially with goals like quitting smoking, people are encouraged to tell others about their plans, to “make themselves accountable” for sticking to them. This feels good, because telling other people about your goals provides the same dopamine boost, the same sense of satisfaction, that the brain would produce after actually meeting the goal in question. The problem is that this makes your brain less “hungry” for that same boost, and takes away one source of motivation which can help you meet your goals. So try not to short-circuit your plans by telling them to other people, at least not prematurely.

The other thing about goals is that there are two kinds, at least. The normal idea of a goal is an achievement-based goal, where it’s about where we get to, where we’re going. The other way of looking at it is a behavioural goal, which isn’t about where we’re headed but about how we get there; it focuses on the journey, not the destination. So an achievement goal would be “I want to write a book.” A more realistic version of this would be “I want to write a book by the end of the year.” A behavioural version of this goal would be “I want to write a thousand words a day.” These behavioural goals are more useful for many people than achievement goals. If you plan to write a book at a specific time then you plan to spend the intervening time writing a certain number of words with a certain amount of regularity. The achievement goal is impossible without your first meeting the behavioural goal. There’s a quote, that “People don’t really decide their future; they decide their habits, and their habits decide their future,” which I find summarizes the whole idea about these two types of goals succinctly.

Behavioural goals are related to the growth mindset I outlined in a previous post, while achievement goals are kind of like the static mindset I talked about. They’re similarly opposed and fall into the same kinds of thinking. Static ideas and achievement based goals do seem to be much more common than the growth mindset and behavioural goals, but are markedly less effective. Another important point is that you should set yourself small, realistic goals to meet today, or tomorrow, or this week. If you give yourself a specific time and make your goals easy, maybe even fun, without setting the bar too high, then you get your brain into the habit of following through and seeking that completion reward, rather than just enjoying the rewarding feeling of having a plan without the need to follow through with it.

The other thing is that even if your goals are behavioural, they can still be unrealistic. Saying “I want to work out every day and never make a mistake and study for two hours every night” is pretty much impossible, and setting yourself up to fail like this makes it less likely that you’re going to succeed in the future. This means there’s something like a meta-level of behavioural goal setting. I want to behave in positive ways which can, over time, improve my understanding and physical and mental fitness, but I also want to accept my mistakes and see them as part of the learning process, rather than evidence that it’s not going to work, and beyond this I also want to be able to slowly and realistically improve the consistency of my behaviour, rather than planning to start a new, high-powered routine immediately and being incredibly disappointed and demotivated if I fail to stick to it after the first week.

So, to get to where you want to be, it might help to plan to behave in ways which lead to success, to de-emphasise specific goals about what grand accomplishments you’re going to make, to give yourself small goals in the short term which are easy for you to meet, to increase the challenging intensity of your goals over time, and to keep the whole thing a secret, at least until it’s well under-way and you’ve already started to receive some of those mental rewards rather than getting all of your satisfaction from the positive ideation itself, and the related affirmation you get from telling your friends.

This is the CDF version of our Christmas Card.

The dimensions  of the Raspberry Pi are 85.60mm x 56mm x 21mm.

A case is a good idea.

This year we have ten students learning calculus and at least half of them will study it next year when they go to university. Engineering, economics and architecture are the most common career paths for our calculus students.

I have been teaching calculus since 1997. During this time, I have had a large enough sample of students to make reliable inferences about skill levels and common biases. In fact student errors are extremely predictable and can be quantified statistically. However, the most interesting thing about teaching is designing a sequence of lessons that will develop their understanding.

Recent changes to NCEA Level 2 have weakened basic knowledge of graphs in a number of students studying year 13 calculus.  The graphs assessment is now an internal, students could use technology to investigate more realistic and engaging scenarios, however the current coverage of graphs is narrower and only marginally more constructive than before and some schools have decided not to teach graphs in year 12.  A similar unwelcome development in year 13 calculus is that some schools don’t teach trigonometry, consequently a number of students are not properly prepared for rigorous tertiary courses like engineering.

Currently all our students are studying integration, but over the last month the first five minutes of their lessons has been used to revise basic graphs and their key features. Calculus is visual and these graphs economically encode many mathematical facts.

The following are the graphs we have been revising. The image and the interactive CDF below were prepared using Mathematica.

If you want to try the CDF you will need to download cdf player.

[WolframCDF source=”http://www.ew.org.nz/wp-content/uploads/2013/09/Blog29-09-2013b.cdf” width=”641″ height=”695″ altimage=”http://www.ew.org.nz/wp-content/uploads/2013/09/Blog29-09-2013b.png” ]